
Share your thoughts, questions, and suggestions in the comments section below! However, even though parallelograms do not have line symmetry, they do have rotational symmetry since any parallelogram, after a rotation of 180 degrees, will result in the exact same image as you started with. Parallelograms have zero lines of symmetry because it is impossible to draw a line through the center of any parallelogram that divides the figure into two equal halves that are mirror images of each other. In today’s lesson, we explored parallelogram lines of symmetry, whether or not they exist, and whether or not parallelograms have any symmetry at all.Īfter reviewing the properties of parallelograms, namely that they are quadrilaterals where the opposite sides and opposite angles are equal, we went on to determine whether or not parallelograms have any line symmetry.īy applying the definition of a line of symmetry, we concluded that, while shapes like squares and rectangles do indeed have lines of symmetry, that parallelograms do not have any lines of symmetry. In the diagram below, you can see that a square has four lines of symmetry, while a rectangle and a rhombus each have only two lines of symmetry. In fact, a shape can have multiple lines of symmetry. If parallelograms do not have lines of symmetry, then why doesn’t a parallelogram have lines of symmetry?įor starters, let's note that a line of symmetry is an axis or imaginary line that can pass through the center of a shape (facing in any direction) such that it cuts the shape into two equal halves that are mirror images of each other.įor example, a square, a rectangle, and a rhombus all have line symmetry because at least one imaginary line can be drawn through the center of the shape that cuts it into two equal halves that are mirror images of each other. What is the number of lines of symmetry in a parallelogram? Now that you understand the key properties and angle relationships of parallelograms, you are ready to explore the following questions: The following diagram illustrates these key properties of parallelograms: And any pair of adjacent interior angles in a parallelogram are supplementary (they have a sum of 180 degrees).
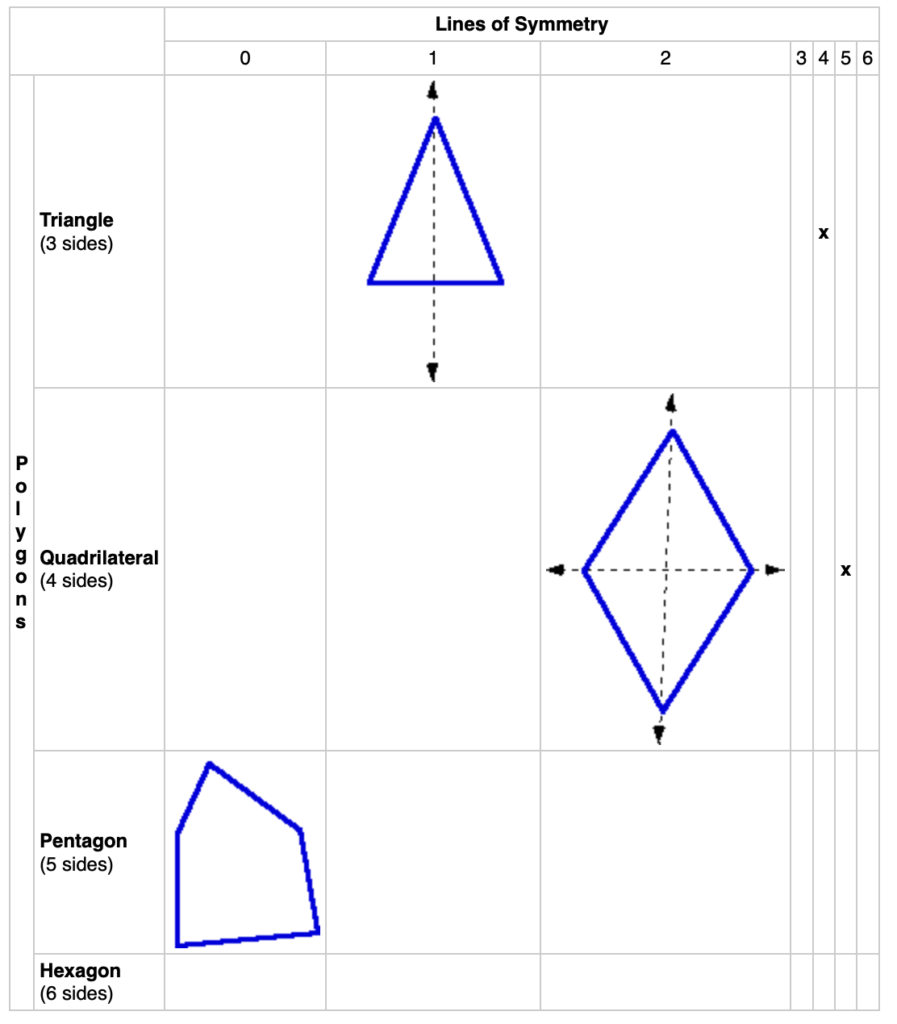
And, if a parallelogram has line symmetry, what would parallelogram lines of symmetry look like (in the form of a diagram).īefore we answer these key questions related to the symmetry of parallelograms, lets do a quick review of the properties of parallelograms: What is a parallelogram?ĭefinition: A parallelogram is a special kind of quadrilateral (a closed four-sided figure) where opposite sides are parallel to each other and have equal length.įurthermore, the interior opposite angles in any parallelogram have equal value. In this post, we will quickly review the key properties of parallelograms including their sides, angles, and corresponding relationships.įinally, we will determine whether or not a parallelogram has line symmetry.
#Line of symmetry free#
The file can be run via the free online application GeoGebra, or run locally if GeoGebra has been installed on a computer.Every Geometry class or course will include a deep exploration of the properties of parallelograms. The file should be considered a draft version, and feedback on it in the comment section is highly encouraged, both in terms of suggestions for improvement and for ideas on using it effectively. This task includes an experimental GeoGebra worksheet, with the intent that instructors might use it to more interactively demonstrate the relevant content material. Students may also accidentally double their answer by counting how many “spokes” go from the center rather than how many complete lines there are. On part (c), students might connect opposite vertices or opposite sides but not both (getting an answer of 2 rather than 4). In all of these cases, it would be very beneficial for students to have scissors and tracing paper so they can trace and cut the shapes out. The fourth figure would have line symmetry if it weren't for the internal lines-this figure should produce a good conversation about what it means for figures to be symmetrical. The tricky thing about part (b) is that all the figures have symmetry, but only two have line symmetry (the other two figures have rotation symmetry). Students are most likely to have difficulty when the lines of symmetry that have a diagonal orientation.

Identifying the lines of symmetry gets increasingly complex for the figures in part (a). Then they can darken the line represented by the fold to reinforce that it is a line of symmetry for their shape. If students are first learning about symmetry, it would be good for them to create their own line-symmetric shapes by folding a piece of paper in half and cutting a shape out. The purpose of this task is for students to identify figures that have line symmetry and draw appropriate lines of symmetry.


 0 kommentar(er)
0 kommentar(er)
